Möbius Strips
Since quite a few works of fiction mention Möbius Strips (click here to see a listing), I am including a description here of this interesting bit of mathematical non-fiction as a resource for anyone who may not have encountered it before.
Topology
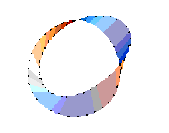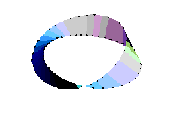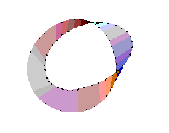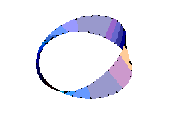Topology is the area of mathematics which studies how different points
on an object (or in a space) are connected to each other. For
example, it is often pointed out that to a topologist a donut and a
tea cup are the same. If you ignore how far apart points are, and
what shape the surface takes (both of which are studied by geometry,
not topology) then the only significant feature about these two
objects is the hole (in the middle of the donut and in the handle of
the tea cup.) This may make topology sound a little bit silly; it
is not!
Surprisingly, there are many unexpected and deep theorems in topology,
and topology is very important in other areas of mathematics like
geometry and even calculus! The easiest example of unexpected
topological results is the "Mobius band", a simple twisted cylinder of
paper with surprising topological properties.
Making a Mobius Band
Take a rectangular piece of paper which is at least twice as long as
it is wide. Laying it on the table in front of you, color the
top-left and bottom-right corners green and color the top-right and
bottom-left corners red. Then roll the paper (with a twist) so that
the two red corners meet each other and the two green corners meet
each other. Fasten it in this shape with tape or glue. You have made
a topological Mobius band (or "Mobius strip"). (It would be best if
you now ignore the "seam" where you have fastened the two ends
together. A perfect band would not have any such break but would be
smooth all around...I just can't figure out how to tell you to make
one of those!)
How Many Sides?
What to do:
Using a crayon, draw a circle on the band.
Pinch the band between your index finger and thumb with your index
finger inside the circle. Now, pull the band between your pinched
fingers until the circle comes around again. Notice that this time
your thumb is in the circle and your index finger is on the "other side".
What does it mean:
The Mobius band only has one side (even
though the paper you made it out of had two). If you try to color
"just one side" of the band with a crayon, you will find that you have
colored the whole thing. It also only has one edge: draw a mark
anywhere on the edge, start your finger at any other point on any edge
(even opposite the mark) and run your finger along the edge. You
will eventually touch the mark.
Cutting Tricks
There are two good tricks you can do with a Mobius band and some
scissors. In each case, the outcome is surprising...unless you
already know some topology in which case you can predict exactly what
will happen!
- Draw a line right down the middle of the band (in the long
direction, that is) and cut along that line (all the way around until
you get back to where you started cutting). What happens? How many
Mobius bands do you have now?
- Cut along a line which always stays exactly one quarter of the
distance from the edge. (Note, you will have to cut twice as far this
time to get back to where you started!) What happens? How many
Mobius bands do you have now? Be careful: not every band is a
Mobius band...some are just ordinary tubes. You can check whether a band
is a Mobius band by checking how many sides it has.
Keep learning
If you find the Mobius band interesting, you should learn more about
its properties (like "non-orientability") and about other topological
objects (like the Klein bottle) by reading a book on topology or
taking a course in topology.
See also: